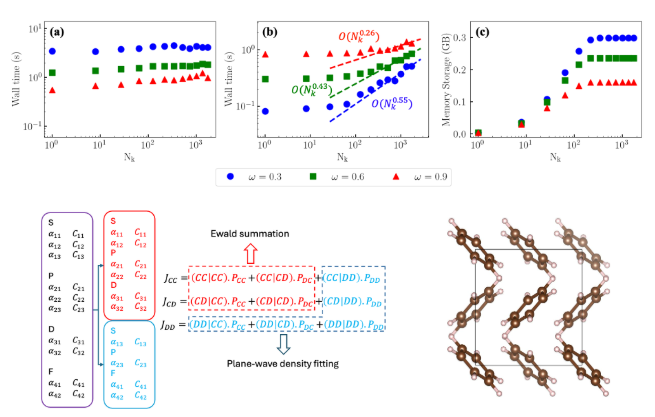
(4)
H. Q. Dinh*, A. Rettig*, X. Feng, J. Lee†
“Efficient All-Electron Periodic Fourier-Transformed Coulomb Method”.
arXiv:2509.16460
You can find Joonho's updated list of publications on his Google scholar page.
(Underlined are names of group members, * denotes equal contribution, † denotes corresponding author.)














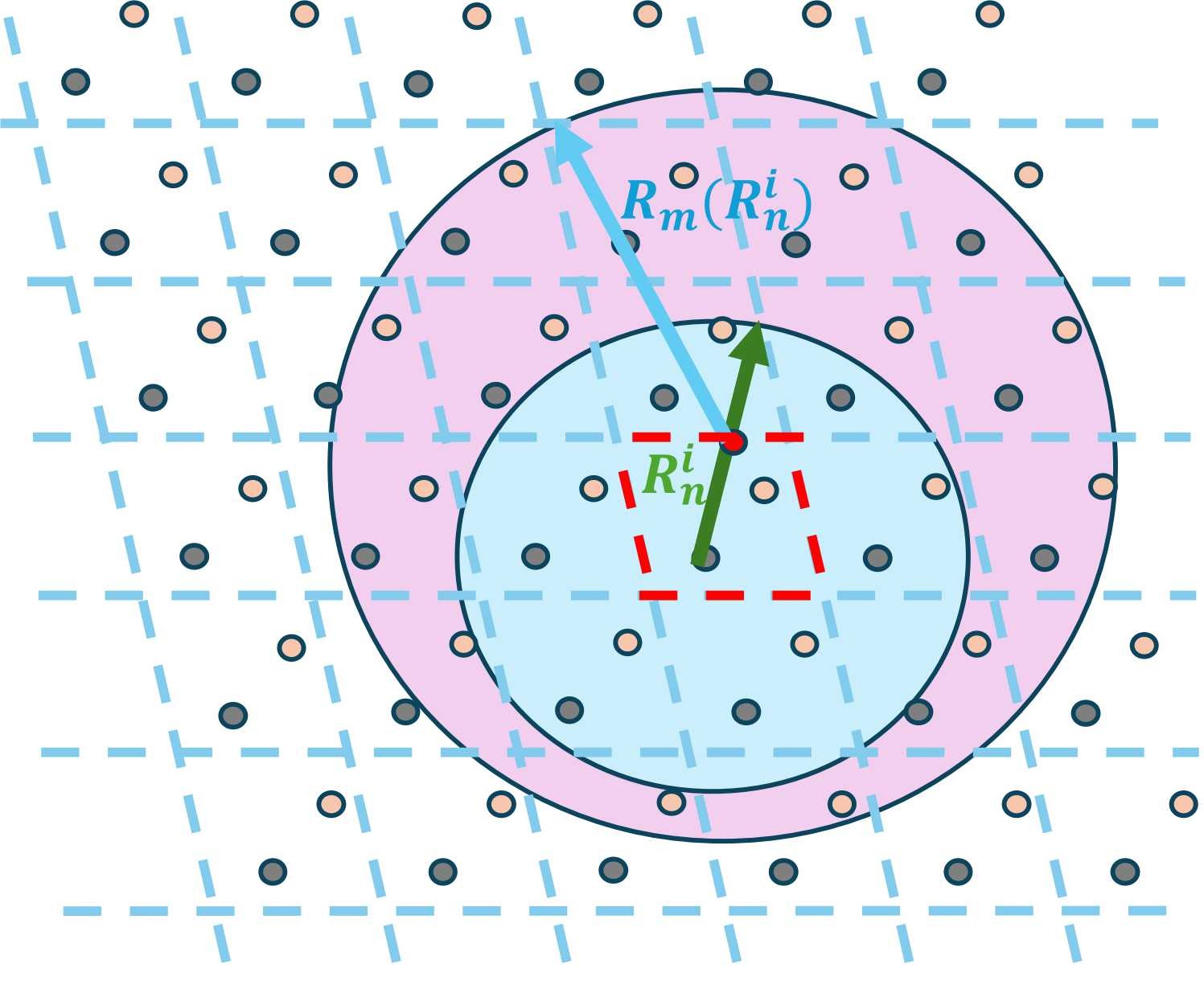


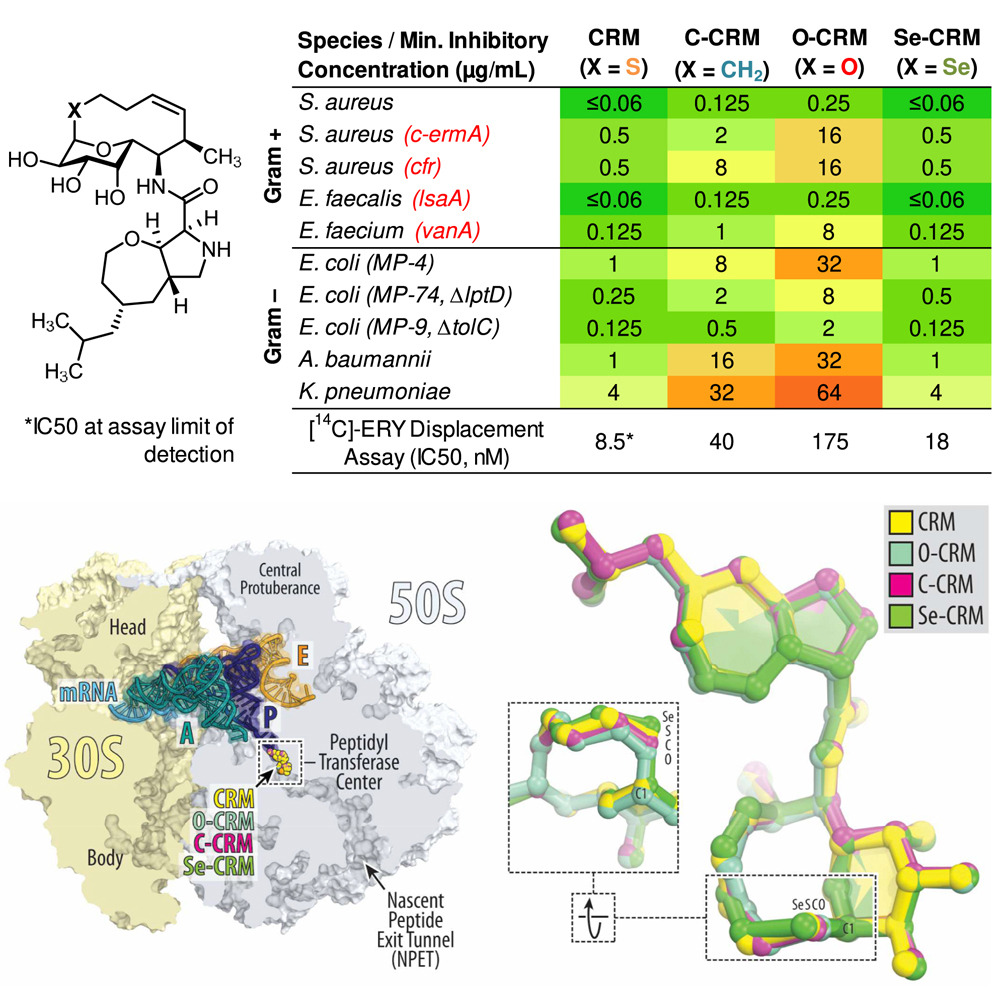

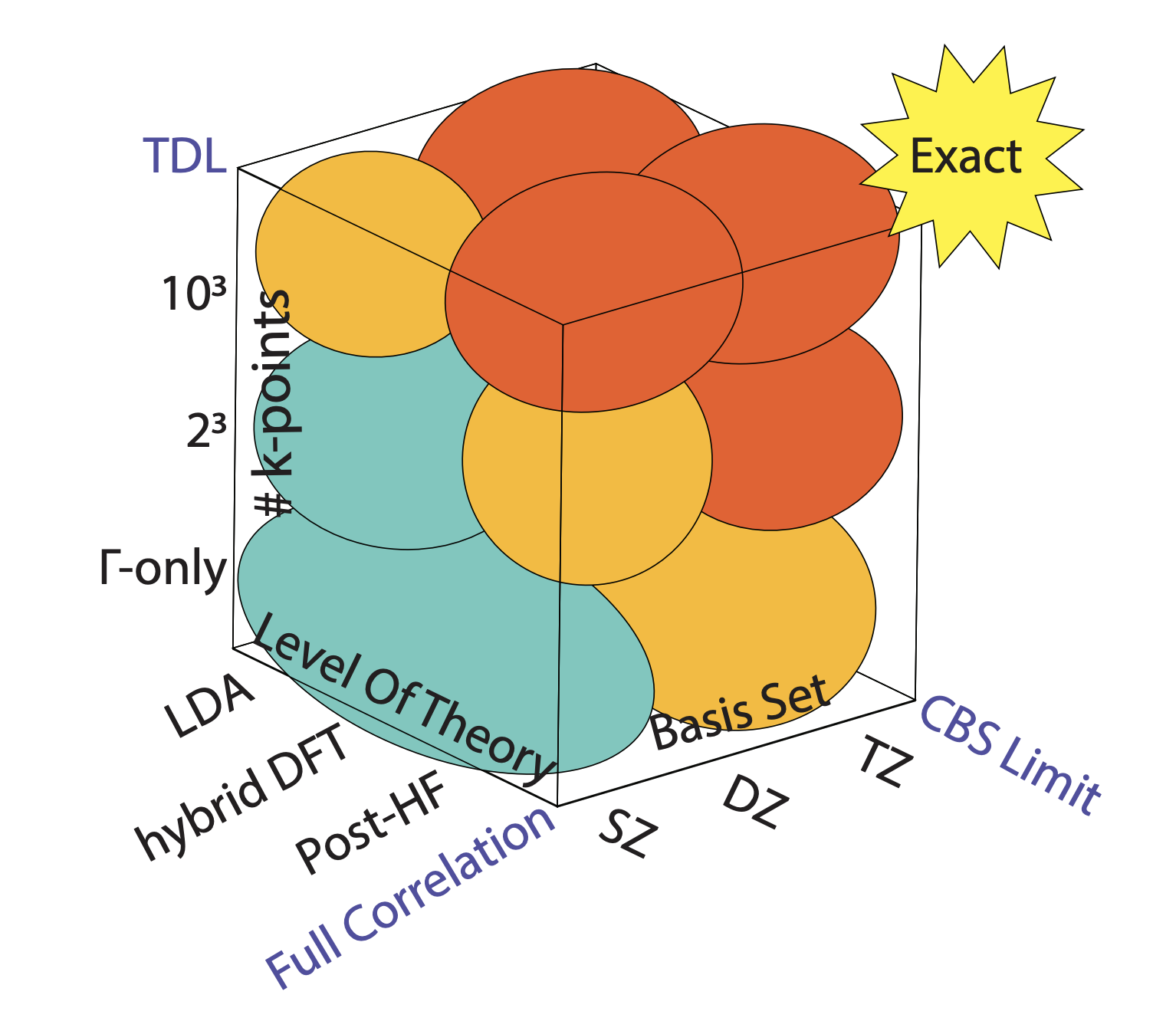

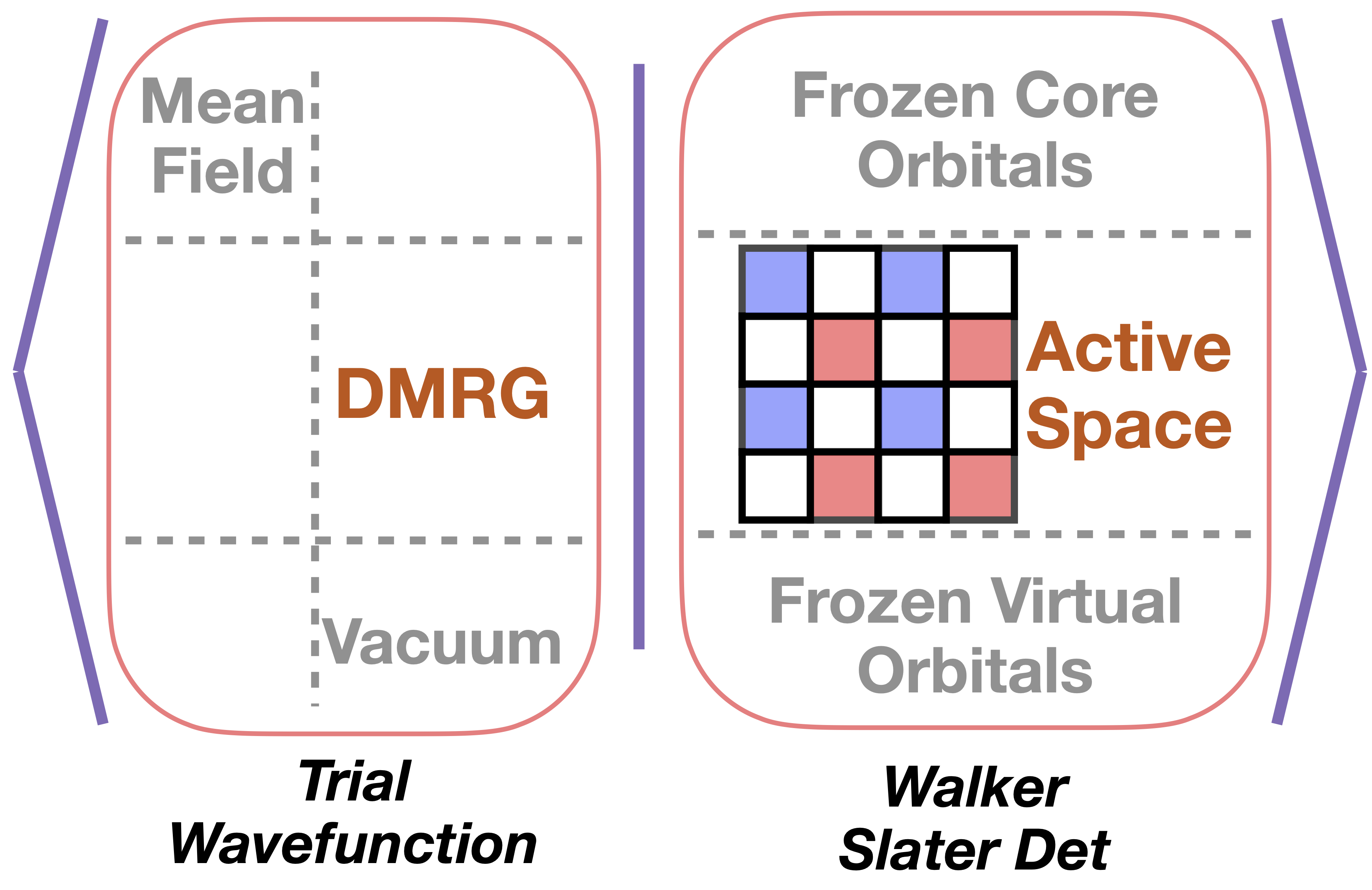



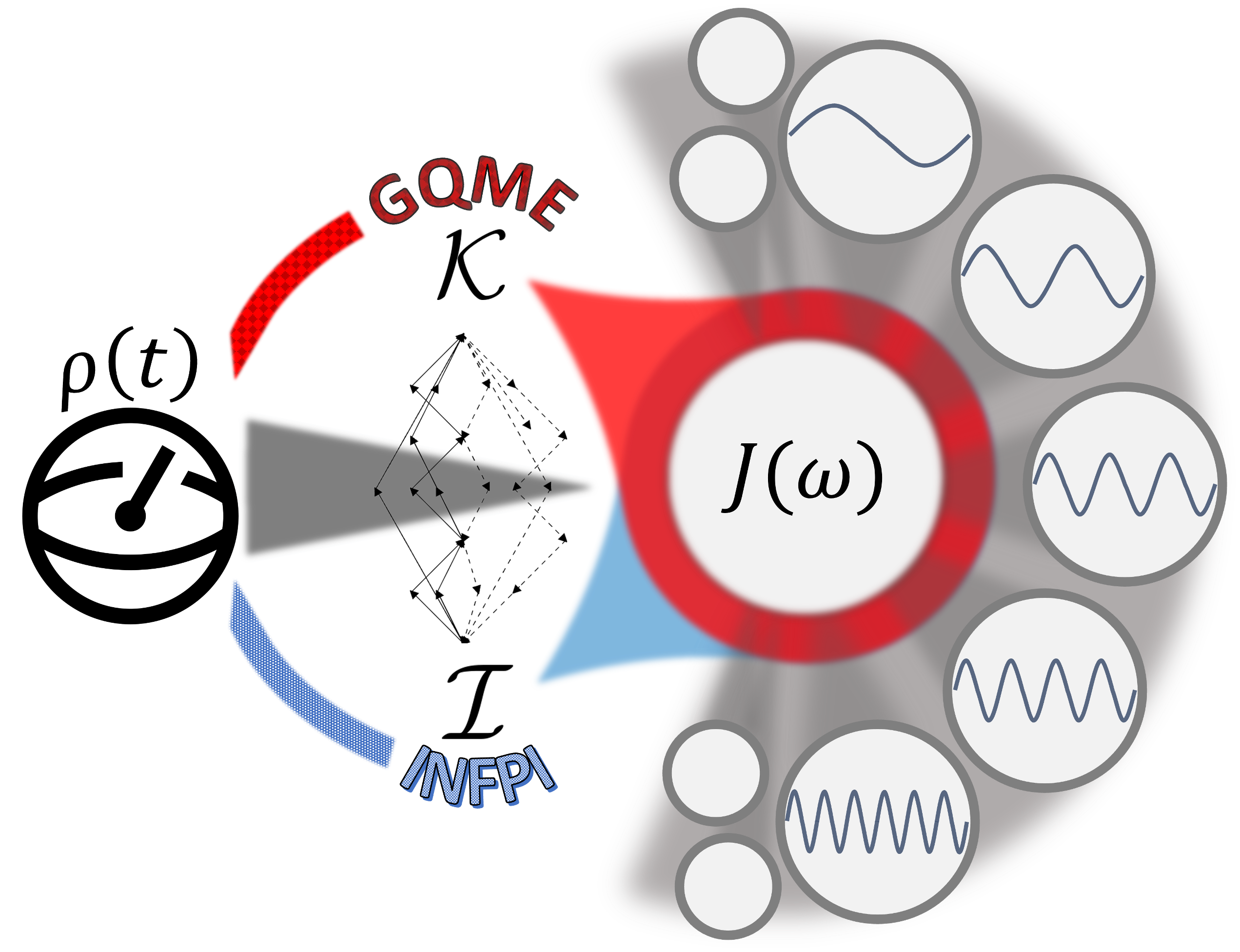

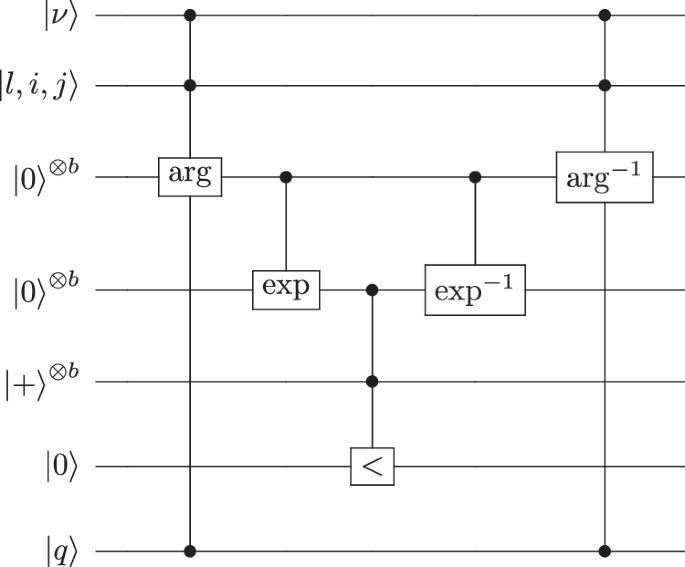

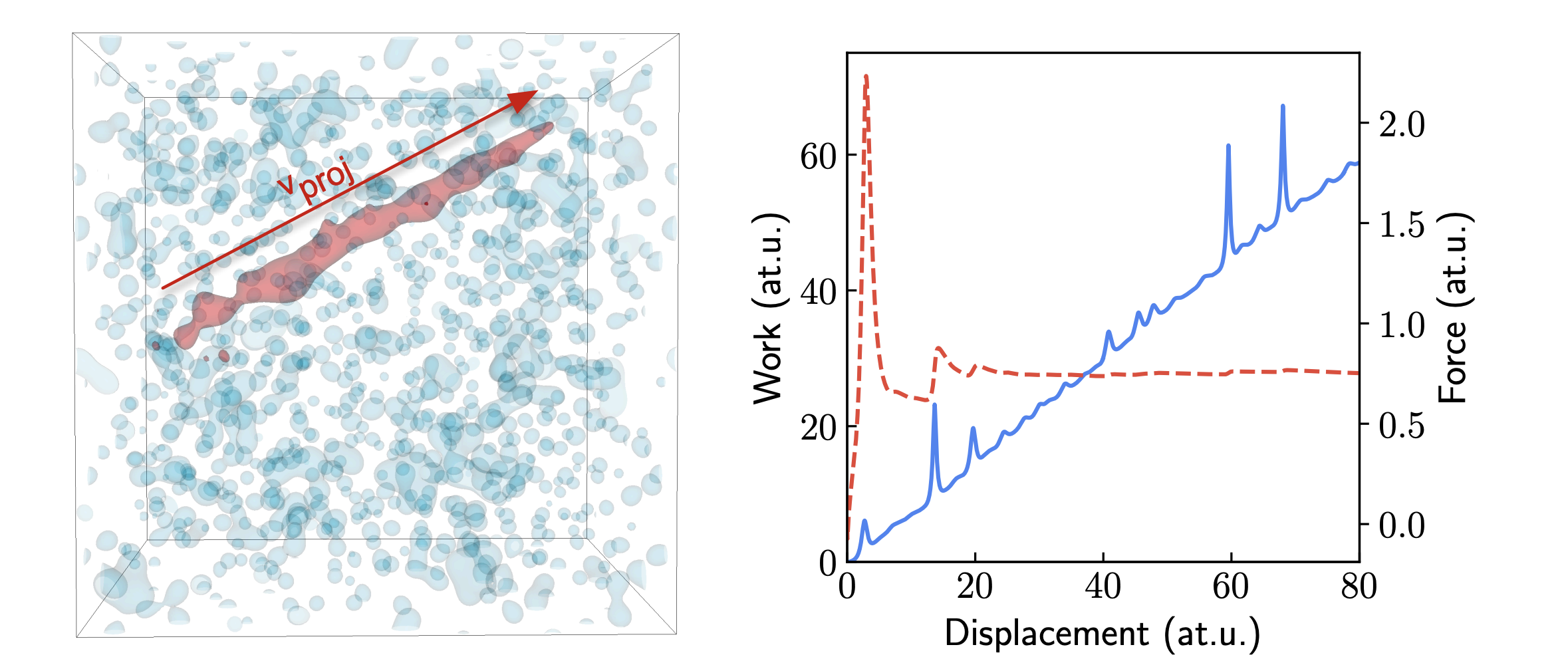



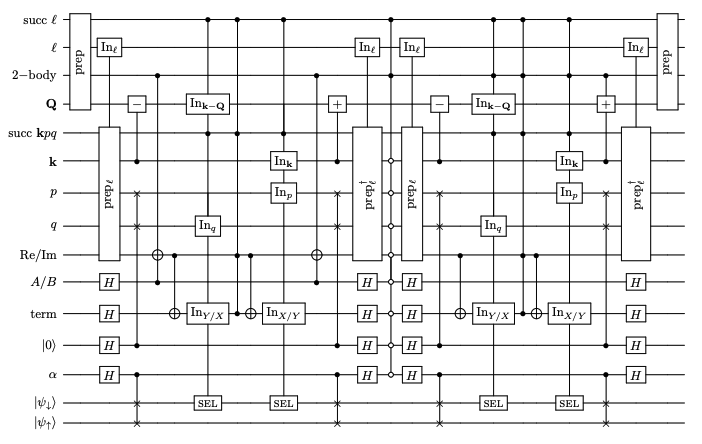

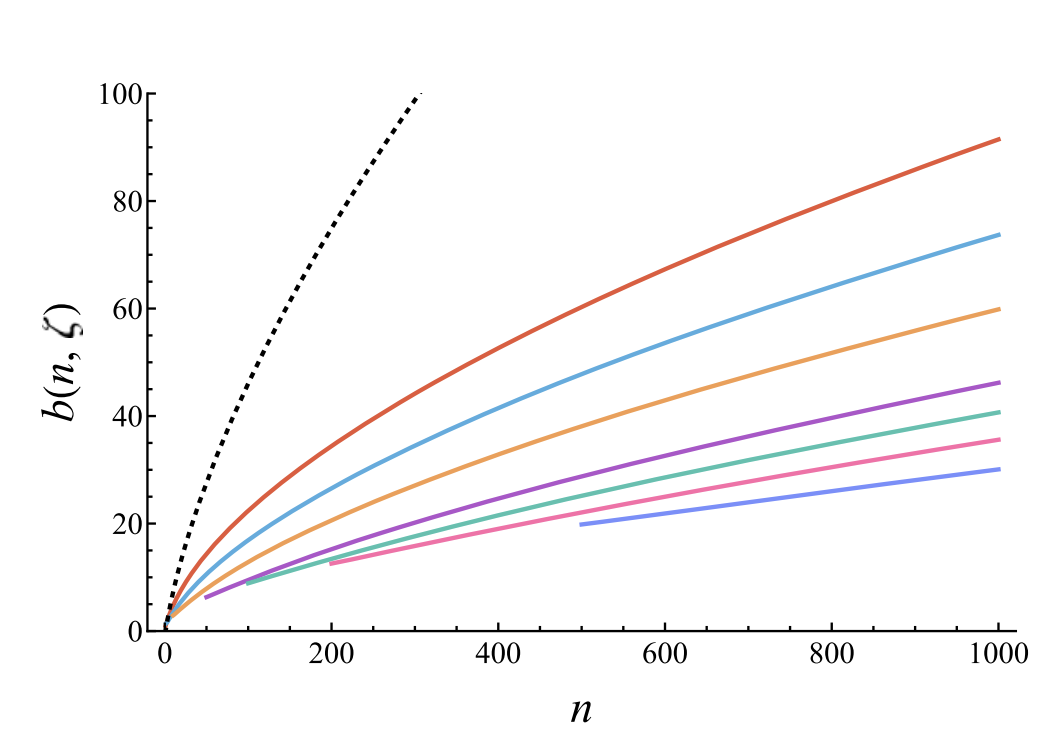

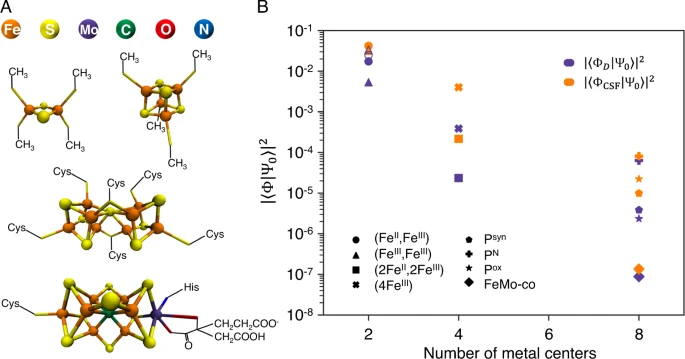



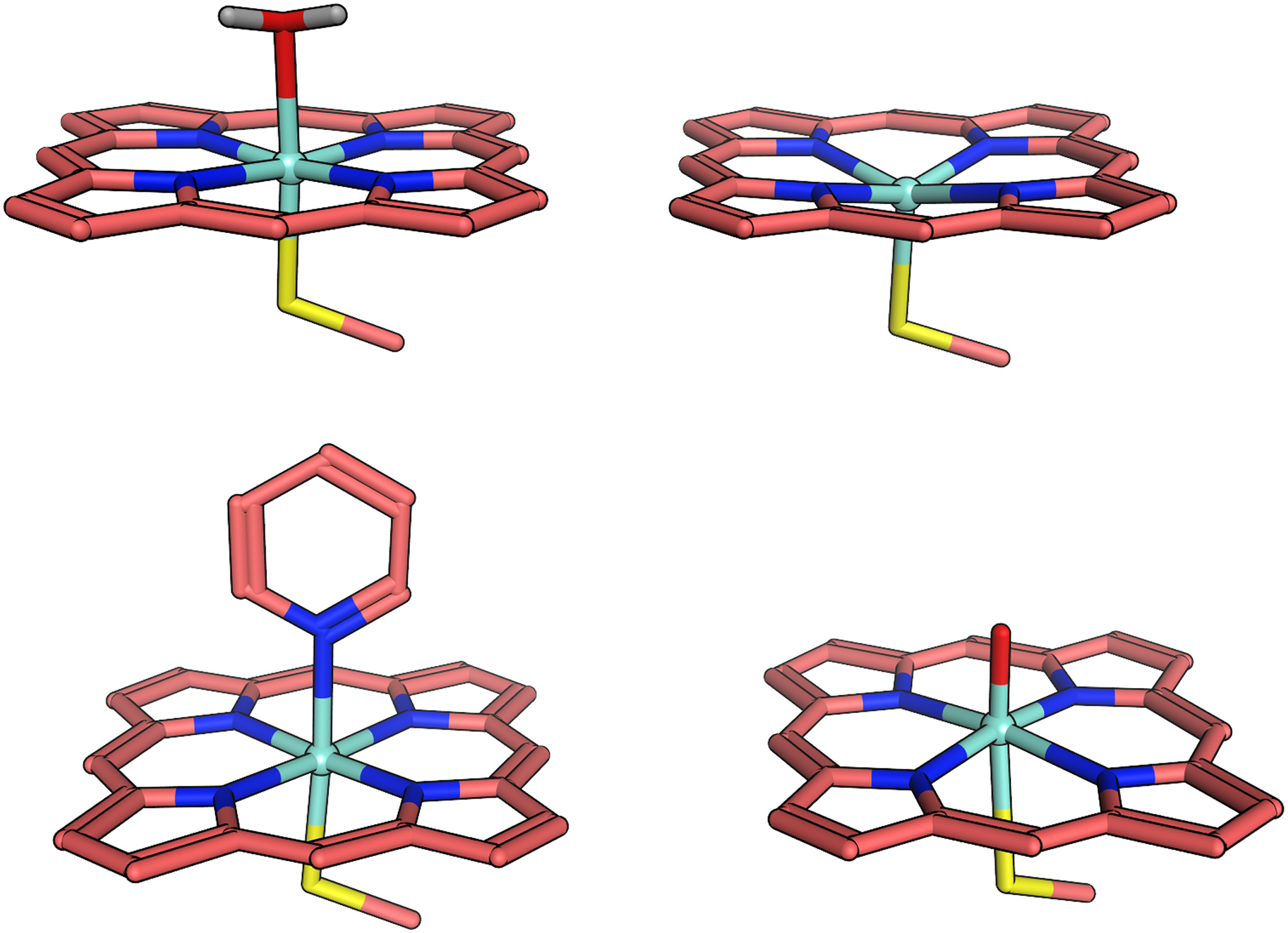

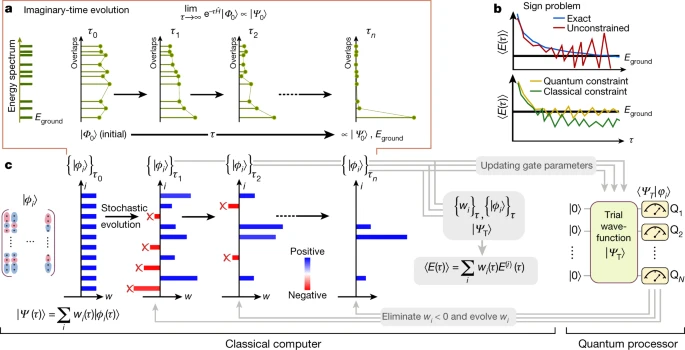



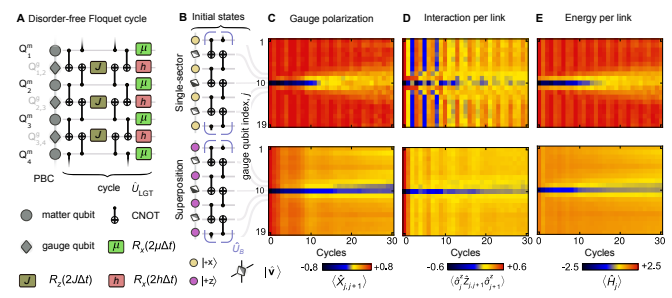

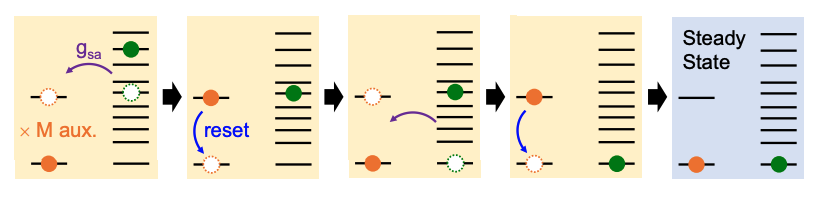

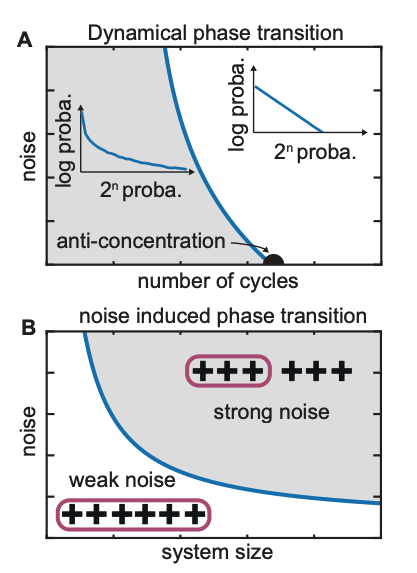





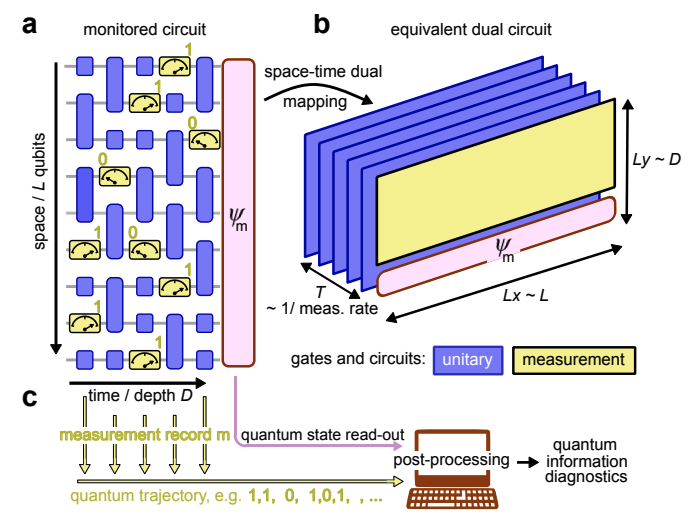



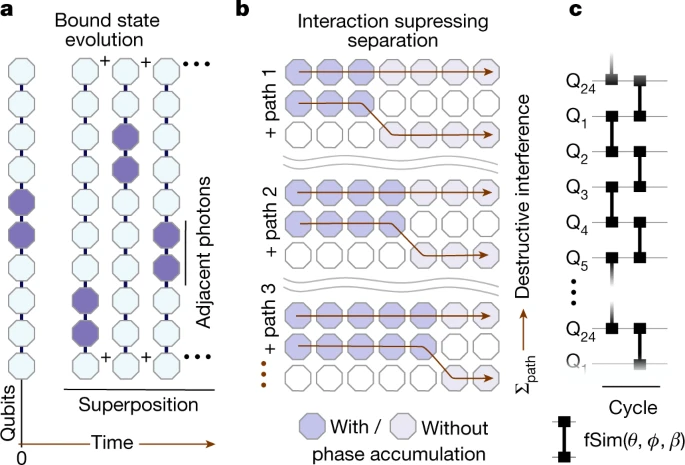

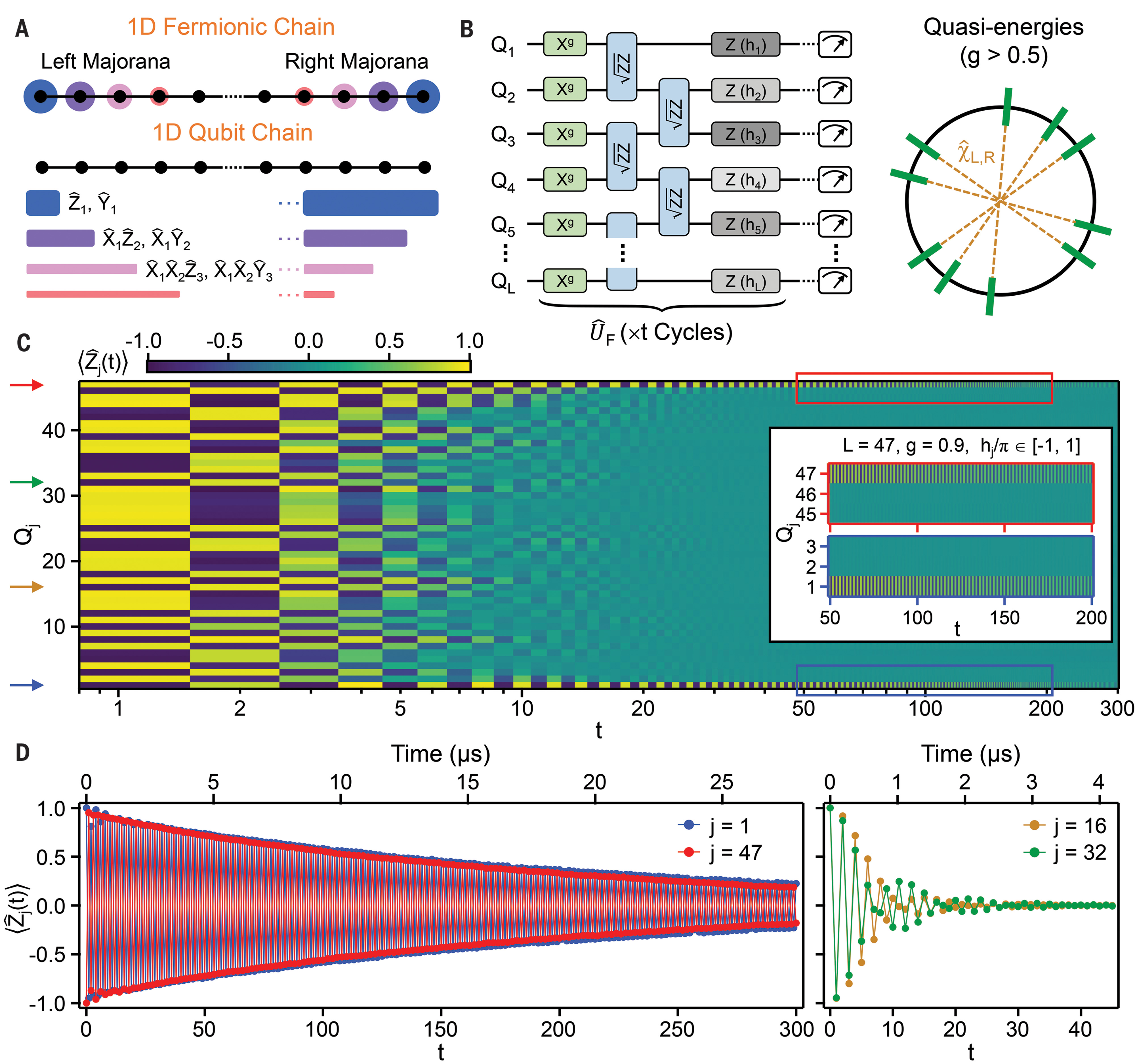







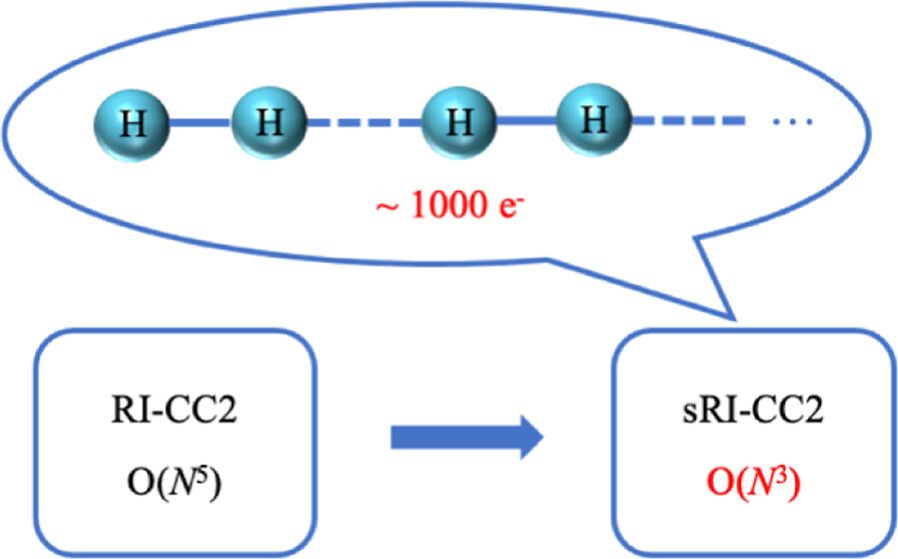









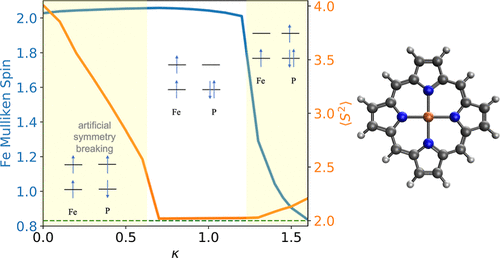

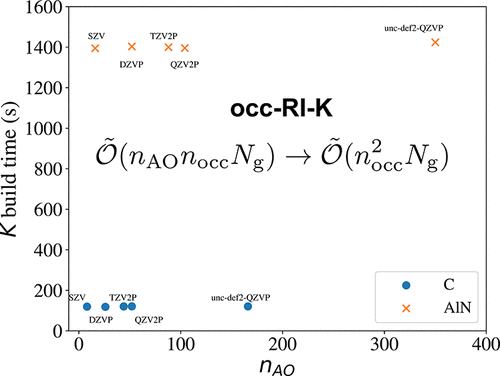




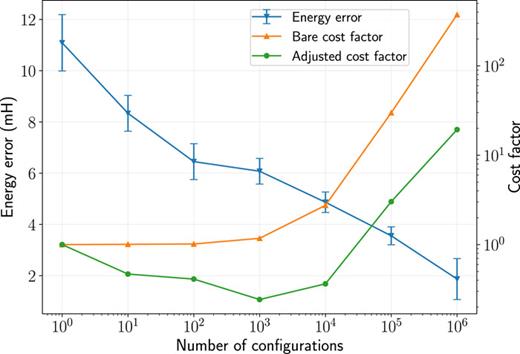

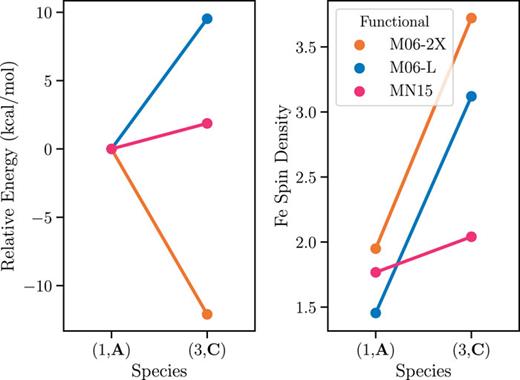



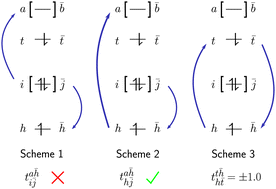

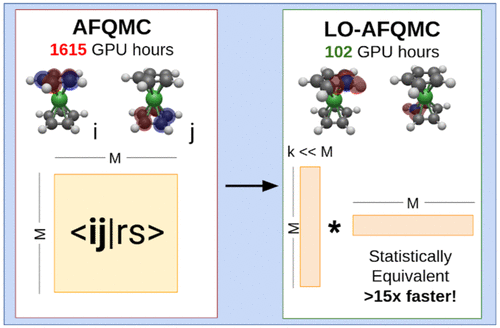

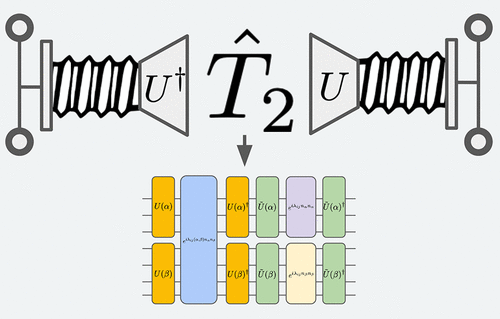



















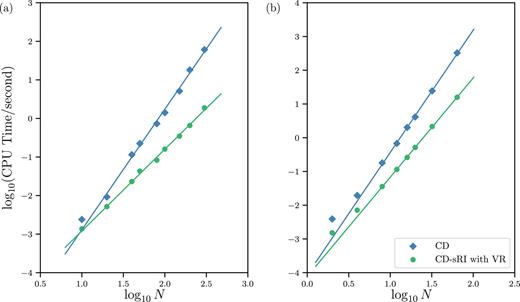

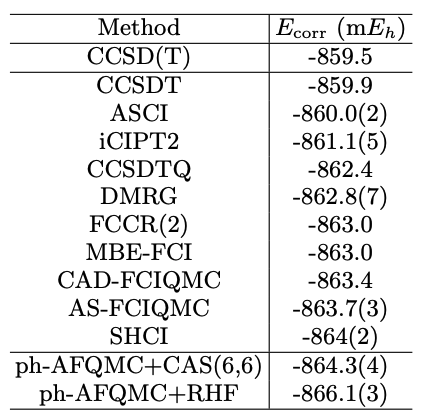

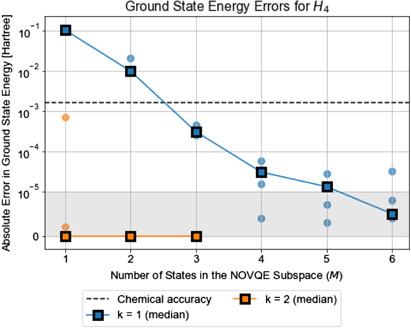

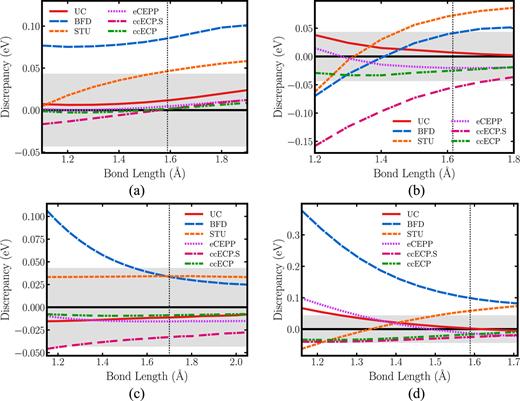

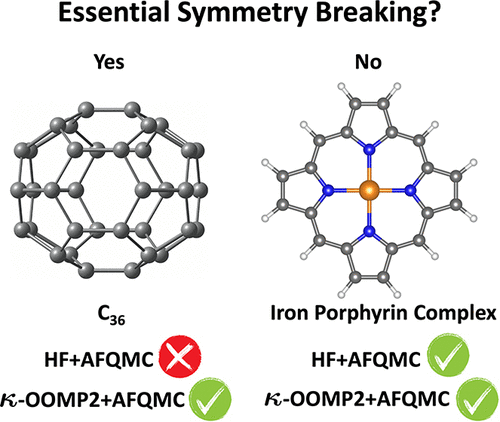





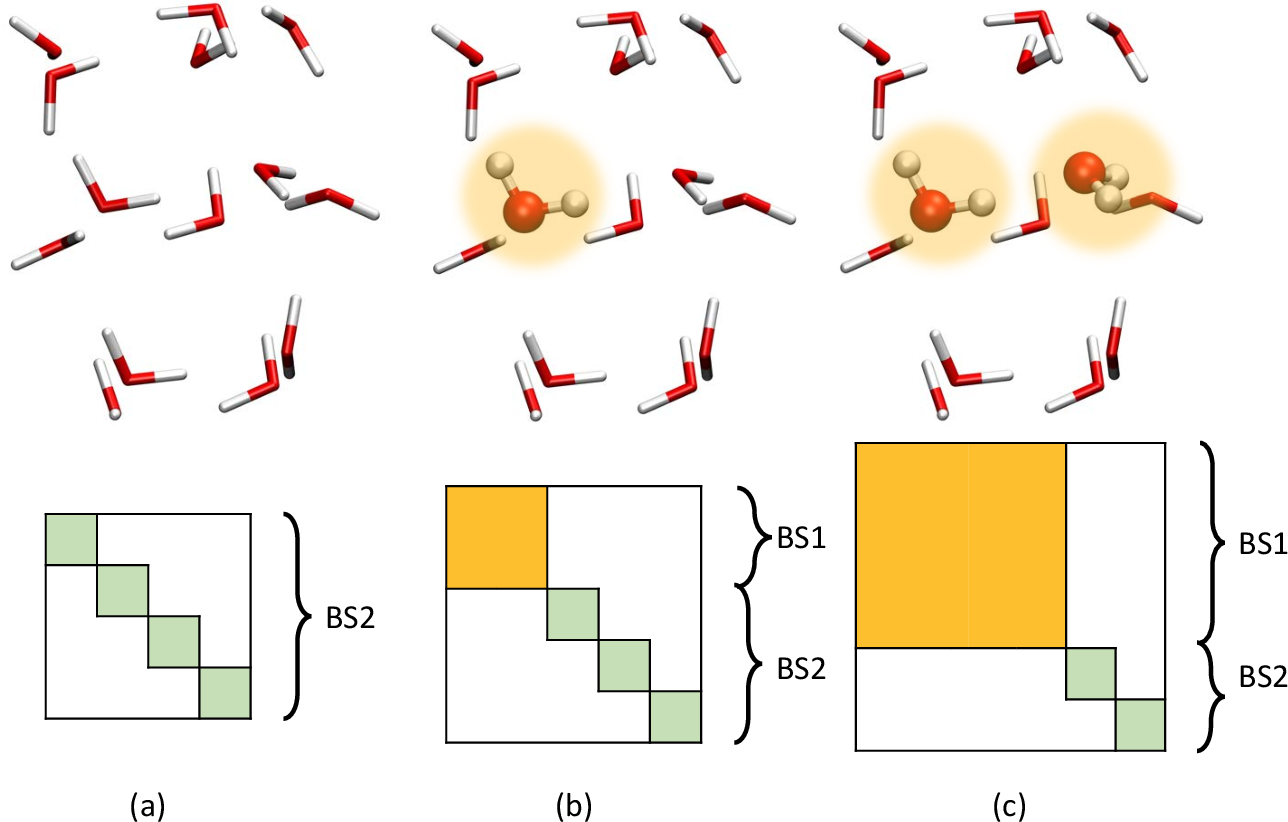



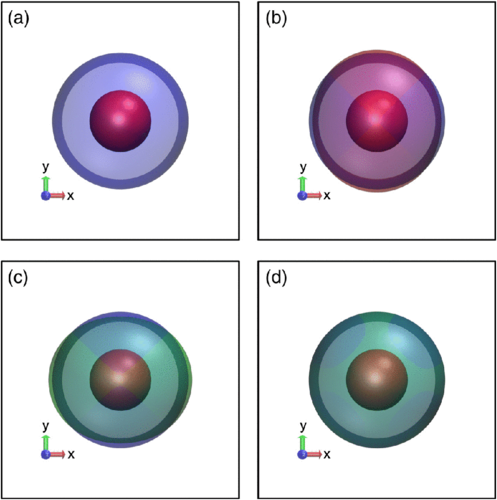

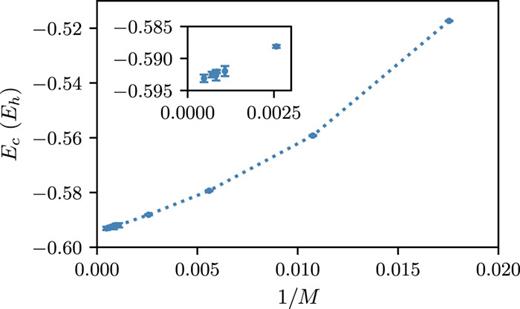






.gif)

.gif)

.jpeg)

.gif)

.gif)

.gif)

.gif)

.jpeg)

.gif)

.png)

.gif)
